kshancock.co.uk

Teaching Math(s) with Worked Examples - some thoughts and experiences

Worked Example Home

Having listened to Michael Pershan (@mpershan on Twitter) talk about using worked examples to teach Mathematics on both the Education Reasearch Reading Room (ERRR) podcast (link here) and the Mr Barton Maths podcast (link here), I ordered his book (link here) and I've been trying it out.

This page contains some of my thoughts, experiences and worked examples over the past half term (Summer Half Term 1 - 2021)

As is always the way with these things - this is my personal interpretation of what I've heard and read.

Using worked examples in lessons to introduce methods:

"Students do not learn from a worked example; students learn when they think actively and deeply about a worked example." M Pershan, 2021

To this end the self-explanation prompts are key, and the hardest part of this, or at least so I've found.

Attempt 1: In which my self-explanation prompts need a lot of work.

After this lesson I realised that the first question was not needed.

As part of the lesson process students explain/have explained the steps in the example and the first question is a repeat of this.

The second question worked to emphasise that you use the perpendicular height rather than any of the other side lengths.

A few students realised that if they were asked for the perimeter they would need all the side lengths, but in retrospect I don't know if it was needed as a question.

I had hoped this question would make student realised that you could halve the 13 and then solve 19.5=6.5h, but this question didn't work at all.

If I did this again I might ask a question related to knowing h but not one of the parallel sides.

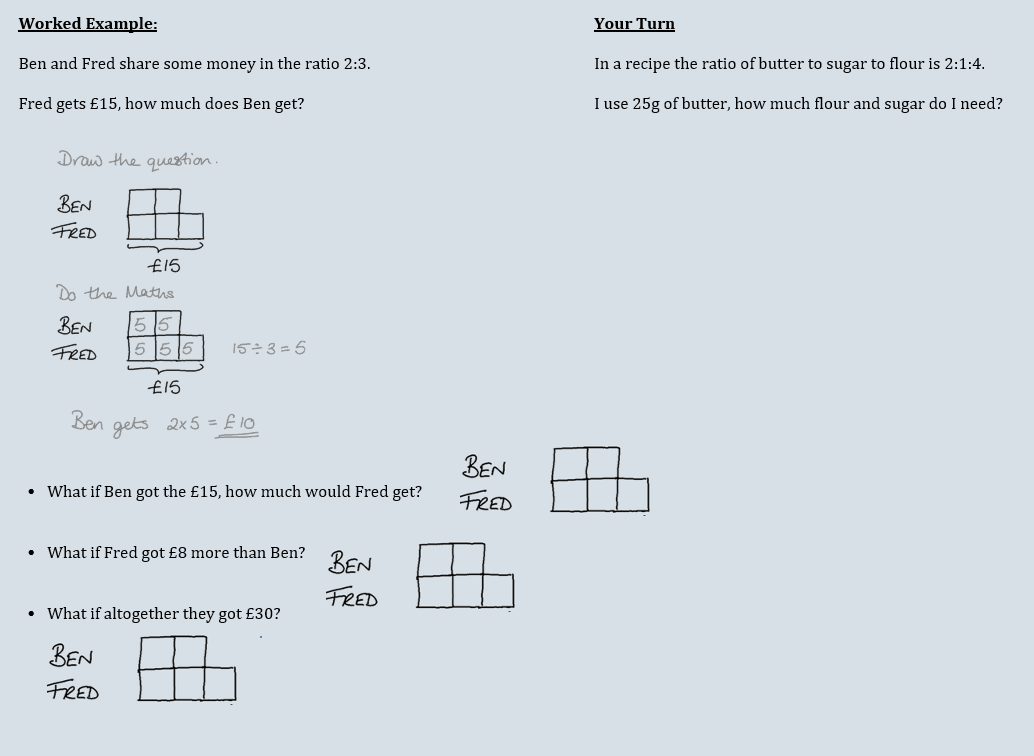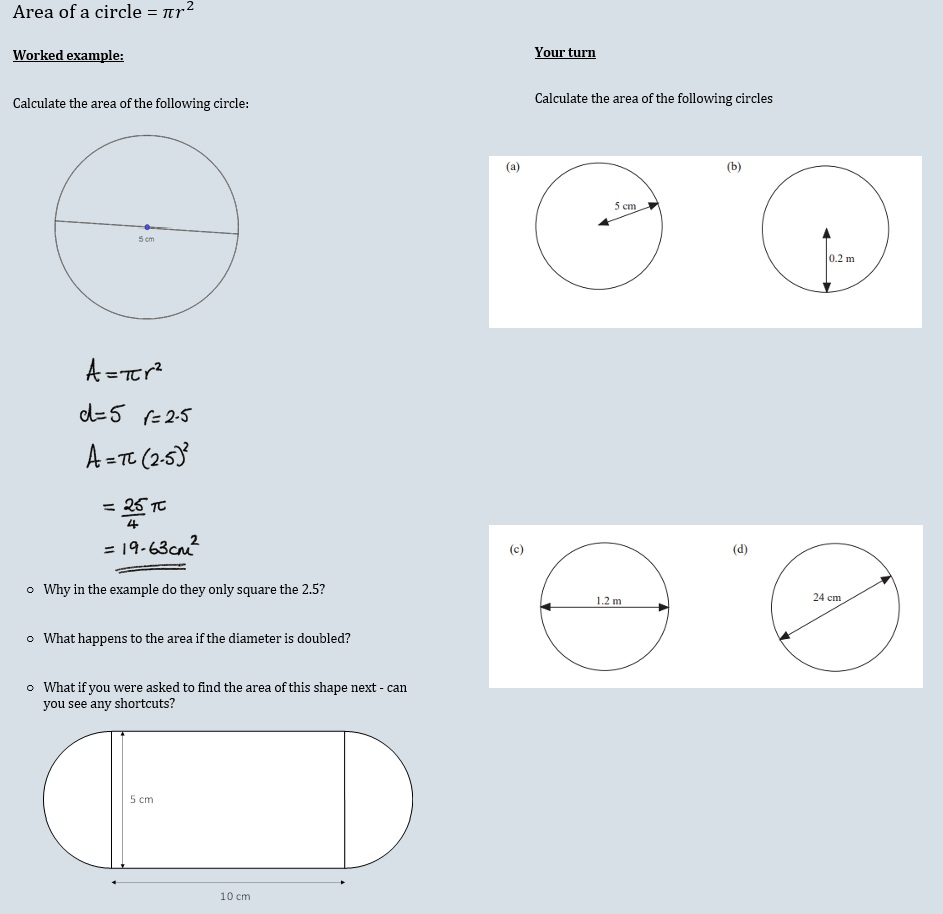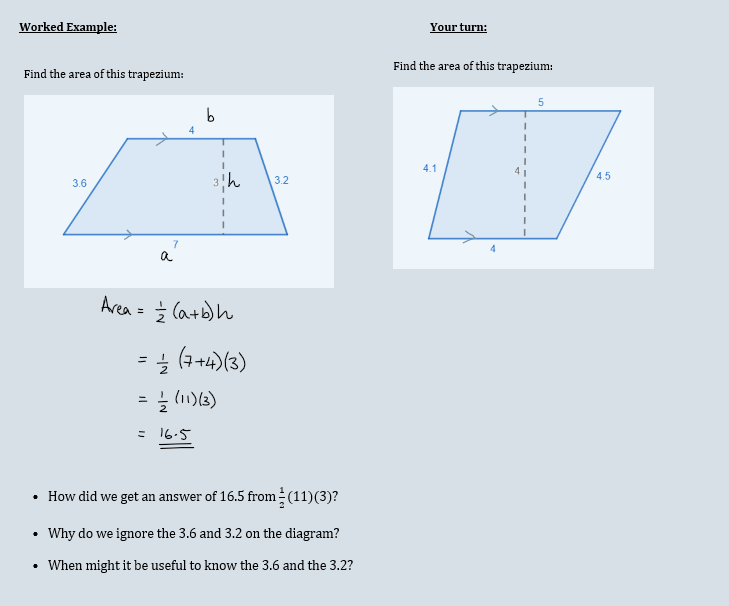
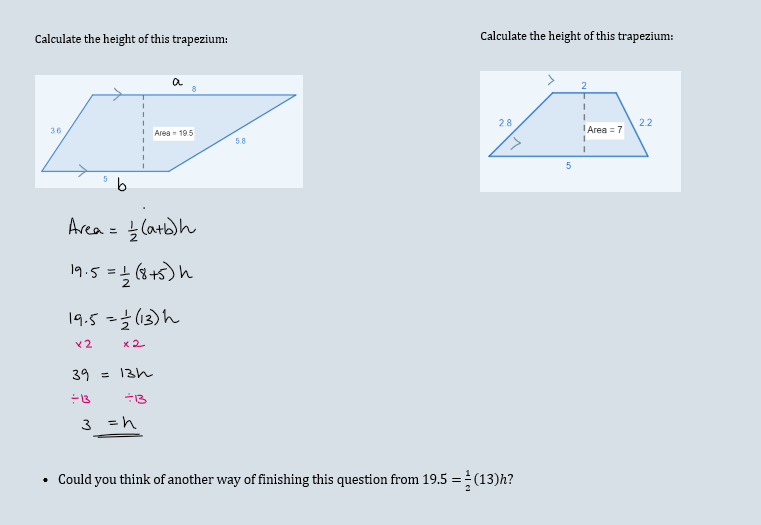
Attempt 2: In which I try not to ask questions about the process, but about the "what if"

An tweet reply from Michael Pershan made me think differently about the self-explanation prompts.

In my own teaching I lately find myself relying a lot on "what if" questions to generate generalizations. As in, "what if instead of x = 2 it had been x = 3" and things of that sort. Ping me if you want to think about an example together?
— Michael Pershan (@mpershan) April 19, 2021

So this time I tried to focus on the "what if"

Attempt 3: In which I'm teaching ratio to a different class, so I get a second go and "what if" plays a big role.

A few weeks later I was teaching the same topic to a different class and I decided to start it differently.

I focused on the "what if" again, but asked a lot more questions.

Students are given paper copies of this and encouraged to write in their answers to the self-explanation prompts.

The ratio examples were harder to write my solution for, because I wanted the students to see the empty boxes so they could see how I then filled them. However, if they were doing this question I wouldn't expect them to draw the boxes twice.

Attempt 4: In which I ask better questions and realise I need more than one "your turn" question to build on these.

This is the latest example from the half term

I realised at this point that only one "your turn" question on the handout was a bit of a waste.

Previously I'd work through an example on the board and then expect the students to complete the "your turn" in their book as their example. After that I'd give them a small selection of basic questions to do before moving onto a different/deeper task.

Since they now have a full worked example to stick in, I can include some of the basic exercise in the "your turn" section.

I felt that with this set of self-explanation prompts I'm starting to make progress in making them think about the example, and how they can use this in harder questions

Conclusions

I've noticed that by using worked examples in this way the students are talking to each other more about the maths that is going on in the examples.

Is it working better than working through the example in real time? Currently, I couldn't say. In times of Covid, everything in the classroom is a bit different, so trialling this in Summer Term 2021 maybe not have been the best idea!

This is just the beginning of the journey and I'll update you when I've had some more chances to use worked examples.